The Time Effect in the Growth Rates Approach

After a very long process our original paper on the growth rates approach was rejected by JEEM about a month ago. I think the referees struggled to see what it added to more conventional approaches. A new referee in the 2nd round hadn't even read the important Vollebergh et al. paper, so it's not surprising they missed how we were trying to build on that paper. That, discussion with my coauthor, Paul Burke, and preparing a guest lecture for CRWF8000 on the environmental Kuznets curve, got me thinking about a clearer way to present what we are trying to do. It really is true that teaching can improve your research!

Vollebergh et al. divide the total variation in emissions in environmental Kuznets curve models into time and income effects:

where G is GDP per capita, E is emissions per capita, and i indexes countries and t time. They point out that the standard fixed effects panel estimation of the EKC imposes very strong restrictions on the first term:

Each country has a constant "country effect" that doesn't vary over time while all countries share a common "time effect" that varies over time. They think that the latter is unreasonable. Their solution is to find pairs of similar countries and assume that just those two countries each share a common time effect.

In my paper on "Between estimates of the emissions-income elasticity" I solved this problem by allowing the time effect to take any arbitrary path in any country by simply not modeling the time effect at all and extracting it as a residual. The downside of the between estimator is that it is more vulnerable to omitted variables bias than other estimators.

We introduced the growth rates approach to deal with several issues in EKC models, one of them is this time effects problem. The growth rates approach partitions the variation in the growth rate of emissions like this:

where "hats" indicate proportional growth rates, and X is a vector of exogenous variables including the constant. The time effect is the expected emissions growth rate in each country when the economic growth is zero. This is a clear definition. The formulation allows us to model the time effect in each individual country i as a function of a set of country characteristics including the country's emissions intensity, legal origin, level of income, fossil fuel endowment etc. I don't think this is that clear in the papers I've written so far. We focused more on testing alternative emissions growth models and, in particular, comparing the EKC to the Green Solow and other convergence models.

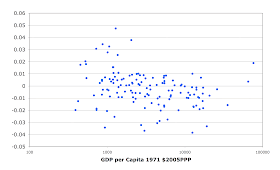
So what do these time effects look like? Here are the time effects for the most general model for the CDIAC CO2 data plotted against GDP per capita in 1971:

Yes, I also computed standard errors for these, but it's a lot of hassle to do a chart with confidence intervals and a continuous variable on the X-axis in Excel.... There is a slight tendency for the time effect to decline with increased income but there is a big variation across countries at the same income level. And here are the results for SO2:

These are fairly similar, but more negative as would be expected. Clearly the time effects story is not a simple one and one that has largely been ignored in the EKC literature.